The De Sitter metric

and its three isotropic forms on the hyperboloid SO(1,4)/SO(1,3)

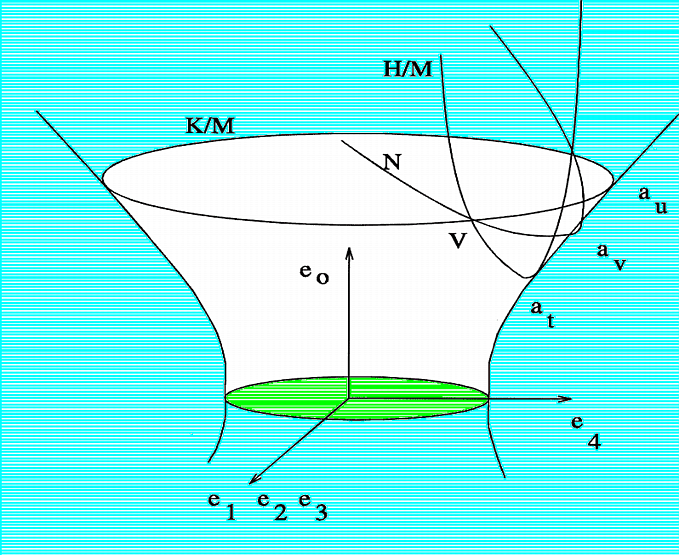
In the De Sitter group G=SO(1,4), let us put K=SO(4), H=SO(1,3), M=SO(3) and N the R^4 sub-group of the Iwasawa decomposition. The restriction to hyperboloid G/H, drawn above, of the metric on R^5, which is invariant by the action of the De Sitter group, gives us the three types of models of universe.

The isometric imbedding of each model of universe is carried out in this De Sitter invariant space R^5.